Local Invariants of Framed Fronts in 3-Manifolds
University of Liverpool, Liverpool, UK Suliman Alsaeed University of Liverpool, Liverpool, UK
Abstract
The front invariants under consideration are those whose increments in generic homotopies are determined entirely by diffeomorphism types of local bifurcations of the fronts. Such invariants are dual to trivial codimension 1 cycles supported on the discriminant in the space of corresponding Legendrian maps. We describe the spaces of the discriminantal cycles (possibly non-trivial) for framed fronts in an arbitrary oriented 3-manifold, both for the integer and mod2 coefficients. For the majority of these cycles we find homotopy-independent interpretations which guarantee the triviality required. In particular, we show that all integer local invariants of Legendrian maps without corank 2 points are essentially exhausted by the numbers of points of isolated singularity types of the fronts.
Keywords
1 Introduction
Vassiliev’s [Vassiliev1994] now classical paper on finite-order invariants of knots was an implementation of Poincaré’s general idea to study properties of generic objects via their degenerations, and thus opened up a new range of problems for singularity theory. Among the first natural questions in this area was a classification of order 1 invariants of planar wave fronts carried out by [Arnold1995], and refined by [Aicardi1997]. A few years later followed Chernov’s classification of similar invariants of fronts on arbitrary surfaces [Tchernov2002].
This paper is on a classification of order 1 invariants of wave fronts in the next dimension, 3. We are assuming the fronts in a 3-manifold $N$ co-oriented, that is, corresponding to Legendrian surfaces in the space $ST^{*}\!N$ of co-oriented contact elements of $N.$ We should notice that we opt for the word ‘framed’ in this context to avoid confusion with co-orientations of discriminantal strata in the space ${\mathcal{L}}={\mathcal{L}}(M,N)$ of all Legendrian immersions of a surface $M$ to $ST^{*}\!N.$
The invariants of generic wave fronts we are looking for are order 1 local, that is, those whose increments in generic homotopies of Legendrian maps are completely determined by diffeomorphism types of the fronts’ local bifurcations. We call such invariants just local since no higher-order invariants will be considered. We make no difference in the exposition between local invariants of Legendrian maps $M\to ST^{*}\!N\to N$ and of framed fronts in $N$ parametrised by $M$: bifurcations of the Legendrian maps are considered in terms of bifurcations of their fronts.
Up to a choice of an additive constant (individual for each connected component of ${\mathcal{L}}$), any numerical local invariant $I$ is defined by its derivative $I^{\prime}=\sum x_{i}X_{i}$, where the $X_{i}$ are discriminantal strata of codimension 1 in ${\mathcal{L}}$, and the $x_{i}$ are the increments of $I$ across them. This linear combination is a trivial codimension 1 cycle in ${\mathcal{L}}.$ Therefore, construction of such linear combinations (without an a priori knowledge of the invariants) splits into two parts:
-
(a)
establishing conditions on linear combinations of the codimension 1 strata to be cycles (we call them discriminantal cycles), and
-
(b)
checking the triviality of the discriminantal cycles.
The first part is a singularity theory task and does not depend on the choice of $M$ and $N$ (except for their orientability) and of a particular connected component of ${\mathcal{L}}(M,N).$ The second part is straightforward if we are able to give an integral (that is, homotopy-free) interpretation of a relevant invariant. However, in more complicated situations knowledge of the fundamental group of a particular connected component of ${\mathcal{L}}(M,N)$ may be required to show that the intersection index of any non-trivial loop in this component with the discriminantal cycle vanishes.
The present paper deals with the singularity-theoretical part (a) and integral cases of part (b) for the fronts, and leaves the topological side of part (b) for further investigations. In our current set-up, we do not assume $M$ oriented (this case will be considered in a forthcoming paper). None of our results depends on a component choice in ${\mathcal{L}}(M,N),$ and, therefore, we always refer to the space ${\mathcal{L}}$ or ${\mathcal{L}}(M,N)$ as a whole, without mentioning its particular components. All invariants are considered up to a choice of additive constants on these components.
Our first main result, Theorem 2.3, states that for framed fronts in any oriented 3-manifold the space of integer discriminantal cycles has rank 6. This space contains a rank 5 subspace which is spanned essentially by the derivatives of the invariants counting the numbers of points of fronts of various stable isolated singularity types. These are triple points of a front, two types of swallowtails, and two types of intersections of cuspidal edges with smooth sheets. A sixth basic discriminantal cycle corresponds to an algebraic count of corank 2 points of Legendrian maps $M\to N$ in generic homotopies. The question of triviality of this cycle is left open. A natural Corollary 2.5 of Theorem 2.3 is a complete classification of integer local invariants of Legendrian maps without corank 2 points: prohibiting homotopies involving corank 2 singularities, we are left exactly with the rank 5 invariant space responsible for the numbers of points of the five isolated singularity types mentioned above.
Our second main result, Theorem 2.6, concerns the $\mathbb{Z}_{2}$ setting of the same problem. It turns out that this time the space of discriminantal cycles has rank 8: in addition to mod2 reductions of the integer cycles, we have cycles counting the parities of direct and opposite self-tangencies of fronts in generic homotopies.
The structure of the paper is as follows.
Section 2 reminds the generalities about Legendrian maps, describes stable singularities of framed fronts under consideration, and states our main results. Section 3 lists discriminantal strata of codimension 1 in ${\mathcal{L}}(M,N).$ Section 4 concentrates on intersection number interpretations of counting corank 2 and opposite self-tangency events in generic homotopies of fronts. Section 5 proves our main theorems via analysis of generic 2-parameter families of fronts. Section 6, the final one, deals with a simplified case of non-framed fronts is oriented 3-manifolds and demonstrates the effect of this simplification on the spaces of dicriminantal cycles and local invariants.
2 Framed Fronts in 3-Manifolds
2.1 General Definitions
We start with recalling a series of standard definitions which may be found, for example, in [Arnold et al.1985] or [Arnold1990].
A contact structure on a $(2n-1)$-dimensional manifold is a non-denerate field of tangent hyperplanes (called contact hyperplanes). Locally, this is a field of zeros of a differential 1-form $\alpha$ (called a contact form), and the non-degeneracy condition is that $\alpha{\wedge}(d\alpha)^{n-1}$ must be a local volume form on the manifold. A manifold equipped with a contact structure is called a contact manifold.
A Legendrian submanifold of a $(2n-1)$-dimensional contact manifold is its $(n-1)$-dimensional submanifold integral for the contact structure.
A fibration $p\colon E^{2n-1}\to B^{n}$ of a contact manifold $E$ over a base $B$ is called Legendrian if all its fibres are Legendrian submanifolds. A composition $M\stackrel{i}{\to}E\stackrel{p}{\to}B$ where $i$ is an embedding of a manifold $M^{n-1}$ into $E$ as a Legendrian submanifold is what is usually called a Legendrian map. However, in this paper we will allow $i$ to be a Legendrian immersion.
The image in $B$ of a Legendrian map $p\circ i$ in called a wave front or just a front.
A contact element of a manifold $N^{n}$ is a hyperplane in the tangent space to $N$ at a point. A contact element with one of its sides in the tangent space distinguished is usually called co-oriented, but we have already mentioned that we will call it framed. The set of all framed contact elements of $N$ is the spherisation $ST^{*}\!N$ of the cotangent bundle of $N.$
A point of $ST^{*}\!N$ is a pair $(\nu,\pi)$ consisting of a point $\nu\in N$ and a framed hyperplane $\pi\subset T_{\nu}N.$ In the tangent space to $ST^{*}\!N$ at $(\nu,\pi)$, consider the hyperplane mapped to $\pi$ under the projection $\rho:ST^{*}\!N\to N.$ The field of all such hyperplanes is the standard contact structure on $ST^{*}\!N,$ and the projection $\rho$ is a Legendrain fibration. The fronts in $N$ of (immersed) Legendrian submanifolds of $ST^{*}\!N$ are naturally framed, at least at their regular points. These are the fronts considered in this paper.
All Legendrian fibrations of the same dimension are locally isomorphic. All through this paper we will be considering the $n=3$ case, and in all our local normal forms, we will be using the traditional local model for this dimension which is the space $J^{1}(\mathbb{R}^{2},\mathbb{R})$ of 1-jets of functions on the plane fibred over $J^{0}(\mathbb{R}^{2},\mathbb{R})\simeq\mathbb{R}_{u}\times\mathbb{R}^{2}_{v,w}$. The contact form here is $\alpha=du-Vdv-Wdw,$ where $V,W$ are coordinates along the fibres of the fibration. The fronts will be framed by the positive $u$-direction. For the orientation of the 3-space we take $du\wedge dv\wedge dw.$
A germ of a Legendrian surface $L\subset J^{1}(\mathbb{R}^{2}_{v,w},\mathbb{R}_{u})$ is defined by its generating family of functions $F(x,v,w)$:
| $L=\{(u,v,w,V,W)|\exists x\colon F_{x}=0,\quad u=F(x,v,w),\quad V=F_{v},W=F_{w}\}.$ |
Sometimes it is convenient to refer to this surface as defined by a generating family $u=F(x,v,w)$ of hypersurfaces. The minimal dimension of the variable $x$ here is the corank of the derivative of the projection $L\to\mathbb{R}^{2}_{v,w}$ at the base point. The smoothness of $L$ requires that the rank of the matrix $(F_{x})_{x,v,w}$ of the second derivatives at the base point must be equal to the dimension of $x$.
An equivalence of two Legendrian maps $L_{j}\to E_{j}\to B_{j},$ $j=1,2,$ is a commutative diagram
| $\begin{array}[]{ccccc}L_{1}&\to&\,\,E_{1}&\to&B_{1}\\ \downarrow&&\varphi\downarrow&&\downarrow\\ L_{2}&\to&\,\,E_{2}&\to&B_{2}\end{array}$ |
in which $\varphi$ is a contactomorphism, and the other two vertical arrows are diffeomorphisms. In terms of local generating families $\Phi(x,u,v,w)=0$ of hypersurfaces this corresponds to the contact equivalence of functions $\Phi$ preserving the fibration $(x,u,v,w)\mapsto(u,v,w)$ (see [Arnold et al.1985]).
2.2 Stratification of a Generic Non-Oriented Framed Front in an Oriented 3-Manifold
The invariants we are going to construct will be expressed in terms of the geometry of the natural stratification of fronts. We shall now remind this stratification. The strata notation follows that of isolated function singularities whose versal deformations serve as generating families for the fronts (see, for example, [Arnold et al.1985] or [Arnold1990]).
A regular point of a generic front ${\mathcal{F}}$ corresponds to an $A_{1}$ singularity of a function in a generating family. Hence the set of all such points will be denoted $A_{1}$.
Irregular points of ${\mathcal{F}}$ are:
| $A_{1}^{2}$ | transversal intersections of two smooth sheets; |
|---|---|
| $A_{1}^{3}$ | same for three sheets; |
| $A_{2}$ | cuspidal edges; |
| $A_{2}A_{1}^{\pm}$ | transversal intersections of edges with regular sheets; |
| $A_{3}^{\pm}$ | swallowtail points. |
The signs in the notations of the last two pairs of singularities distinguish between different framing choices as shown in Fig. 1.
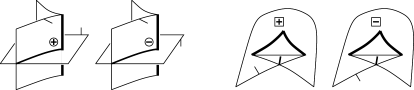
Local generating families of hypersurfaces for the one-component singularities are:
| $A_{1}\colon u=x^{2},\quad A_{2}\colon u=x^{3}+vx,\quad A_{3}^{\pm}\colon\pm u=% x^{4}+vx^{2}+wx.$ |
2.3 Local Invariants
In what follows $M$ will always be a non-oriented compact surface, and $N$ an oriented 3-manifold, both without boundaries. Denote by ${\mathcal{L}}={\mathcal{L}}(M,N)$ the space of all Legendrian maps $M\looparrowright ST^{*}\!N\to N$, where the first arrow is a Legendrian immersion and the second the canonical projection. Maps whose fronts have more complicated singularities than those described above form the discriminantal hypersuface $\Xi$ in ${\mathcal{L}}.$ For example, if $M\looparrowright ST^{*}\!N$ is not an embedding, then the corresponding Legendrian map is in $\Xi.$
Consider connected components of ${\mathcal{L}}{\setminus}\Xi.$ A numerical invariant is a way to assign numbers to each of them. Along a generic path in ${\mathcal{L}},$ the values of an invariant change at the moments of discriminant crossings.
Definition 2.1.
We say that an invariant is local of order 1 (or just local, for short) if every increment of the invariant is completely determined by the diffeomorphism type of the local bifurcation of the front at the crossing.
Considering invariants whose value set is different from $\mathbb{Z}_{2}$, we must have the discriminant locally co-oriented.
A local invariant $I$ defines its derivative $I^{\prime}=\sum_{i}x_{i}X_{i},$ where the $X_{i}\subset\Xi$ are the strata of codimension 1 in ${\mathcal{L}}$ we are able to distinguish for the needs of Definition 2.1, and the $x_{i}$ are the local increments of $I$ along generic paths in ${\mathcal{L}}$ crossing the $X_{i}$ in the co-orienting direction. On the other hand, $I$ is defined by $I^{\prime}$ on each connected component ${\mathcal{L}}_{j}$ of ${\mathcal{L}}$ up to a choice of ‘a constant of integration’, that is, up to an arbitrary choice of the value of $I$ at a non-discriminantal base point in ${\mathcal{L}}_{j}$.
Since the total increment of $I$ along any loop in $L_{j}$ vanishes, the derivative $\sum_{i}x_{i}X_{i}$ must be a trivial codimension 1 cycle in ${\mathcal{L}}_{j}$. The vanishing of the total increment on contractible loops (that is, the derivative being a cycle, maybe non-trivial) is equivalent to its vanishing on small loops in ${\mathcal{L}}$ around codimension 2 strata of the discriminant. Finding the relevant cyclicity constraints on the increments $x_{j}$ is the problem on which we concentrate in this paper. As it was said in Sect. 1, cycles of the form $\sum_{i}x_{i}X_{i}$ will be called discriminantal.
One of the ways to establish the triviality of a cycle $I^{\prime}=\sum_{i}x_{i}X_{i}$ is to find an integral (that is, path-independent) interpretation of its antiderivative $I$ in terms of the geometry of individual fronts.
Example 2.2.
It is clear that the number of isolated singularities of ${\mathcal{F}}$ of a
particular type is a local invariant. We distinguish five such
invariants:
$I_{t}$
the number of triple points $A_{1}^{3}$;
$I_{s_{\pm}}$
the numbers of positive and negative swallowtails;
$I_{c_{\pm}}$
the numbers of $A_{2}A_{1}^{\pm}$ points.
Another obvious local invariant for fronts in $\mathbb{R}^{3}$ or $S^{3}$ is $I_{\ell}$ the self-linking number of the oriented framed link defined by the cuspidal edge of ${\mathcal{F}}$ (as shown in Fig. 2).

In Sect. 4.1 we will introduce yet another candidate for local invariants, $I_{\Sigma^{2}},$ counting an algebraic number of corank 2 points of Legendrian maps in homotopies. We do not have its integral interpretation: we only know its derivative which may happen to be a non-trivial cycle in ${\mathcal{L}}.$
2.4 Classification of the Discriminantal Cycles and Invariants
All statements in this section refer to any compact surface $M$ and oriented 3-manifold $N.$
The first main result of this paper is
Theorem 2.3.
The space of integer discriminantal cycles in ${\mathcal{L}}(M,N)$ has rank 6. Its basis is formed by the derivatives of the invariants
| $I_{t},\quad(I_{s_{+}}\pm I_{s_{-}})/2,\quad(I_{c_{+}}+I_{c_{-}})/2,\quad I_{% \Sigma^{2}},\quad(2I_{s_{+}}+I_{c_{+}}-I_{c_{-}}-6I_{\Sigma^{2}})/4.$ |
This implies
Corollary 2.4.
The space of integer local invariants of framed fronts in $N$ parametrised by $M$ has rank either 6 or 5.
The exact value of the rank here depends on $I^{\prime}_{\Sigma^{2}}$ being or not being a trivial cycle.
Let ${\mathcal{L}}_{1}\subset{\mathcal{L}}(M,N)$ be the set of all Legendrian maps without corank 2 points. Discriminantal cycles in ${\mathcal{L}}_{1}$ do not contain any summands of $I^{\prime}_{\Sigma^{2}}.$ Our proof of Theorem 2.3 yields
Corollary 2.5.
The space of integer discriminantal cycles in ${\mathcal{L}}_{1}(M,N)$ has rank 5. Its basis is formed by the derivatives of the invariants
| $I_{t},\quad(I_{s_{+}}\pm I_{s_{-}})/2,\quad(I_{c_{+}}+I_{c_{-}})/2,\quad(2I_{s% _{+}}+I_{c_{+}}-I_{c_{-}})/4.$ |
Respectively, these five invariants form a basis of the space of all integer local invariants on ${\mathcal{L}}_{1}(M,N)$.
One of the simplest events in generic one-parameter families of fronts is tangency of two smooth sheets. For framed fronts, we have two types of such self-tangencies: direct when the framings of the sheets at the touching point coincide, and opposite when they do not. We denote by $I^{\prime}_{dir}$ and $I^{\prime}_{opp}$ the derivatives of the $\mathbb{Z}_{2}$-valued functions counting the parities of the events in homotopies.
In the $\mathbb{Z}_{2}$ case we have
Theorem 2.6.
The space of mod2 discriminantal cycles in ${\mathcal{L}}(M,N)$ has rank 8. Its basis is formed by $I^{\prime}_{dir},I^{\prime}_{opp}$ and the reduced basic derivatives of Theorem 2.3.
Prohibition of corank 2 points in homotopies cuts here the rank down to 7 and excludes $I^{\prime}_{\Sigma^{2}}$.
Depending on how many of the linear combinations of the mod2 discriminantal cycles $I^{\prime}_{dir},I^{\prime}_{opp}$ and $I^{\prime}_{\Sigma^{2}}$ turn out to be trivial, we have
Corollary 2.7.
The space of $\mathbb{Z}_{2}$-valued local invariants of framed fronts in $N$ parametrised by $M$ has rank at least 5 and at most 8.
3 Codimension 1 Bifurcations
To prove our main results, we first of all need a description of all codimension 1 discriminantal strata in ${\mathcal{L}}(M,N).$ Therefore we will now list all generic 1-parameter families of our Legendrian maps, in terms of the local bifurcations of their fronts. We co-orient the strata in ${\mathcal{L}}$ wherever we can do this by local means. As long as only corank 1 singularities are concerned, the fronts in 3-manifolds do not differ locally from the critical value sets of maps between 3-manifolds. Therefore, in that part, our list is not too far from the relevant part of the list from [Goryunov2013] but has a crucial difference in the strata decorations.
3.1 Multi-Germs
We start with the bifurcations involving more than one local component of a front.
Following the notations introduced for singularities of a generic front, the notations of the bifurcations below are self-explanatory, with $T$ used for the tangency of the participating strata of a front. Letters $e$ and $h$ distinguish between elliptic and hyperbolic versions of similar bifurcations. Letter $r$ stays for the number of faces of the bounded region appearing after the bifurcation, which are framed outward the region. If there is no such bounded region, we make a special comment on the meaning of $r$. The figures illustrate only one particular value of $r$ from the range, or one particular choice of the sign. We use the orientation of the cuspidal edges as defined in Figure 2. We co-orient a discriminantal stratum in ${\mathcal{L}}$ by the direction of the shown sequence of the events in the bifurcation. In some cases we show only the ‘positive resolutions’ of the fronts and indicate by arrows how one of the local components has been moved during the bifurcations.
So, we have (see Figure 3):
$A_{1}^{4,r}$
$r=2,3,4$, intersection of four smooth sheets.
The pre-bifurcation tetrahedral region has $4-r$ faces framed
outwards. Therefore, the $r=2$ stratum $A_{1}^{4,2}$ is not
co-orientable in ${\mathcal{L}}$.
$TA_{1}^{3,r}$
$r=0,1,2,3$, three smooth sheets are pairwise
transversal to each other, but the line of intersection of any two
of them is tangent to the third sheet at the moment of bifurcation.
$TA_{1}^{2,e,r}$
$r=0,1,2$, elliptic tangency of two smooth
sheets.
$TA_{1}^{2,h,r}$
$r=0,1$, same, but hyperbolic. We write $r=1$
if the sheets are framed to the same side, and $r=0$ if the framings
are opposite. For $r=1$, the stratum is not co-orientable in ${\mathcal{L}}$.¡br¿
The strata $TA_{1}^{2,e,r}$ and $TA_{1}^{2,h,r}$ are exactly those
responsible for the direct ($r=1$) and opposite ($r=0,2$)
self-tangencies mentioned in Sect. 2.4.
$A_{2}A_{1}^{2,\pm,\pm}$
cuspidal edge meets the intersection of
two smooth sheets. The two signs are the signs of the two $A_{2}A_{1}$
points after the bifurcation, in the order in which the points lie
locally on the cuspidal edge if we follow the edge orientation.
$A_{2}^{2,e,\pm}$
two edges meet face-to-face. During the
bifurcation the crossing sign of the two local oriented edges
changes. The sign in the notation of the stratum is the crossing
sign after the bifurcation.
$A_{2}^{2,h}$
one of the edges is overtaking the other. The
crossing sign of the two edges after the bifurcation is positive.
$A_{3}^{\pm}A_{1}^{\pm}$
a smooth sheet passes through a
swallowtail. The meaning of the $A_{1}$ sign is clear from the figure.
$TA_{2}A_{1}^{e,\pm}$
cuspidal edge becomes tangent to a smooth
sheet so that the two local components of ${\mathcal{F}}$ do not intersect
before the bifurcation. The two cases differ by the framing of the
smooth sheet.
$TA_{2}A_{1}^{h,\pm}$
the hyperbolic version of the previous.
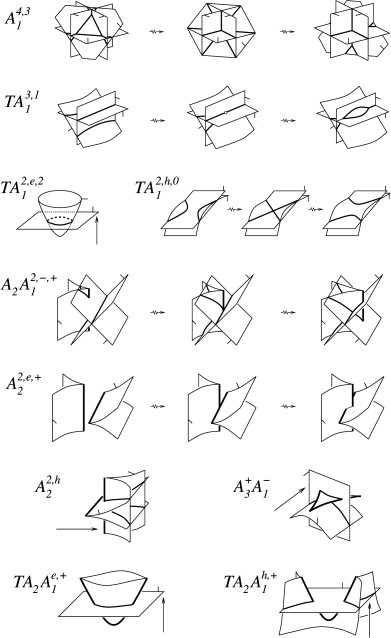
3.2 Uni-Germs
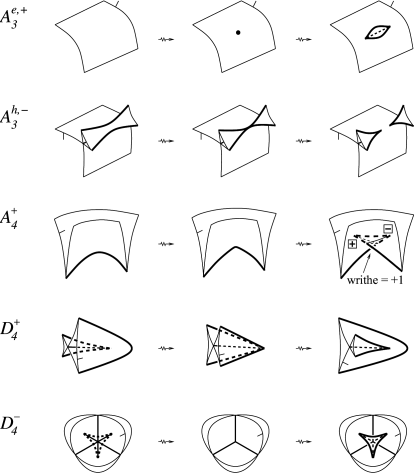
Our normal forms for generic 1-parameter uni-germ transformations follow [Arnold1976]; [Zakalyukin1983], and their illustrations in Fig. 4 correspond to [Arnold1990].
The sign of the real parameter $\lambda$ in the local normal forms for the generating families of hypersurfaces co-orients the strata in ${\mathcal{L}}$. Following what was said about the local normal forms in Sect. 2.2, the tangent plane to the $\lambda=0$ front at the origin is $u=0$ framed by the positive $u$-direction.
| $A_{3}^{e,\pm}$ | $\pm u=x^{4}+(v^{2}-\lambda)x^{2}+wx,$ birth of cuspidal lips, with the swallowtails of the sign in the notation. |
|---|---|
| $A_{3}^{h,\pm}$ | $\pm u=x^{4}+(\lambda-v^{2})x^{2}+wx,$ a beaks bifurcation on the edge. |
| $A_{4}^{\pm}$ |
$\pm u=x^{5}-\lambda x^{3}+vx^{2}+wx,$ here the sign is the local crossing number of the edge after the bifurcation. It coincides with the sign of the two appearing $A_{2}A_{1}$ points. |
| $D_{4}^{\pm}$ |
$u=\pm x^{2}y+y^{3}-\lambda y^{2}+wy+vx,$ the
swallowtails after the bifurcations are positive. This co-orientation of the $D_{4}^{\pm}$ strata coincides with that by the increase of the negative signature of the $A_{3}$ singular points in the generating families. |
Altogether, we have identified 35 codimension 1 strata in ${\mathcal{L}}$, two of which, $A_{1}^{4,2}$ and $TA_{1}^{2,h,1}$, are not co-orientable.
3.3 Counting Isolated Singularities of Fronts
It will be convenient for us to use sums of the strata differing only in certain indices in their notations. In such cases we will omit the corresponding signs or letter and assume that the summation is done across the whole range of the omitted symbols, for example:
| $A_{4}=A_{4}^{+}+A_{4}^{-},\quad TA_{2}A_{1}=TA_{2}A_{1}^{e,+}+TA_{2}A_{1}^{e,-% }+TA_{2}A_{1}^{h,+}+TA_{2}A_{1}^{h,-}.$ |
To avoid confusion to which such abbreviations may lead in some cases, we will also use the notations similar to
| $A_{3}^{e/h,+}=A_{3}^{e,+}+A_{3}^{h,+}\quad{\rm and}\quad A_{3}^{e/h,\pm}=A_{3}% ^{e/h,+}+A_{3}^{e/h,-}.$ |
Lemma 3.1.
The derivatives of the numbers of points of isolated singularity types of generic fronts and of the edge self-linking number are
| $\displaystyle I_{s}/2=(I_{s_{+}}+I_{s_{-}})/2$ | ${\displaystyle:}A_{3}^{e/h,\pm}+A_{4},$ | ||
| $\displaystyle\widehat{I}_{s}/2=(I_{s_{+}}-I_{s_{-}})/2$ | ${\displaystyle:}A_{3}^{e/h,+}-A_{3}^{e/h,-}+D_{4}^{+}+3D_{4}^{-},$ | ||
| $\displaystyle I_{c}/2=(I_{c_{+}}+I_{c_{-}})/2$ | ${\displaystyle:}2A_{2}^{2,e}+A_{3}A_{1}+TA_{2}A_{1}+A_{4},$ | ||
| $\displaystyle\widehat{I}_{c}/2=(I_{c_{+}}-I_{c_{-}})/2$ | ${\displaystyle:}2A_{2}^{2,e,+}-2A_{2}^{2,e,-}+2A_{2}^{2,h}+A_{4}^{+}-A_{4}^{-},$ | ||
| $\displaystyle I_{t}$ | ${\displaystyle:}2TA_{1}^{3}+2A_{2}A_{1}^{2}+A_{3}A_{1},$ | ||
| $\displaystyle I_{\ell}$ | ${\displaystyle:}2A_{2}^{2,e,+}-2A_{2}^{2,e,-}+2A_{2}^{2,h}+A_{4}^{+}-A_{4}^{-}.$ |
In particular, we see that in $\mathbb{R}^{3}$ or $S^{3}$, up to an additive constant, $I_{\ell}=(I_{c_{+}}-I_{c_{-}})/2.$
4 The Two Invariants
We give here details of possible local invariants appearing in theorems of Sect. 2.4.
4.1 Corank 2 Points in Homotopies
Within the lists of Sects. 3.1 and 3.2, the $\lambda=0$ members of the $D_{4}^{\pm}$ families are the only Legendrian maps having rank 0 at the origin. We will now express an algebraic number of such events in generic homotopies of fronts as an intersection number. The construction will imply that
| $I^{\prime}_{\Sigma^{2}}=D_{4}^{+}+D_{4}^{-}$ |
for the possible local invariant $I_{\Sigma^{2}}$ mentioned in Sects. 2.3 and 2.4.
Every contact hyperplane of a contact structure is a symplectic linear space, with the symplectic form $d\alpha$ if $\alpha$ is the contact form. Moreover, the tangent space to a Legendrian submanifold at a point is a Legendrian subspace in the contact hyperplane. This provides a fibration
| $\phi\colon{\mathcal{E}}\to ST^{*}\!N^{3},$ |
with a fibre $\Lambda_{2}$, the Lagrangian grassmannian of non-oriented Lagrangian planes of symplectic $\mathbb{R}^{4}$.
A family of Legendrian immersions $i_{t}\colon M\looparrowright ST^{*}\!N,$ $t\in[0,1],$ lifts to a family of embeddings $\widetilde{i}_{t}\colon M\to{\mathcal{E}},$ $m\mapsto T_{i_{t}(m)}i_{t}(M),$ $i_{t}=\phi\circ\widetilde{i}_{t}.$ Consider:
-
•
a 3-film ${\mathcal{Z}}\subset{\mathcal{E}},$ the union of the images of the embeddings $\widetilde{i}_{t},$ $t\in[0.1],$
-
•
the section ${\mathcal{V}}\subset{\mathcal{E}}$ of vertical Lagrangian planes, that is, the field of the kernels of the derivative of the projection $\rho\colon ST^{*}\!N\to N.$
In general position, ${\mathcal{Z}}$ and ${\mathcal{V}}$ meet at isolated non-boundary points of ${\mathcal{Z}}$, and $\phi$ projects these points exactly to the points of the Legendrian surfaces $i_{t}(M)$ at which their individual projections $\rho$ to the base $N$ have rank 0.
Our aim is to define an intersection number $\langle{\mathcal{V}},{\mathcal{Z}}\rangle$ in ${\mathcal{E}}$, that is, to assign signs to points of ${\mathcal{V}}\cap{\mathcal{Z}}.$ Consider one of these points, $z_{0}=\widetilde{i}_{t_{0}}(m_{0}),$ and the local trivialization $J^{1}(\mathbb{R}^{2}_{v,w},\mathbb{R}_{u})\times\Lambda_{2}$ of ${\mathcal{E}}$ near it, with $\phi(z_{0})=i_{t_{0}}(m_{0})$ being the origin in the first factor for which we will be using the coordinate description given in Sect. 2.1. Projection onto the second factor gives us a map germ $\pi\colon({\mathcal{Z}},z_{0})\to\Lambda_{2},$ and reduces the task to assigning a sign to $\pi.$ We will call $\pi(z_{0})$ the vertical point of $\Lambda_{2}.$
Lemma 4.1.
Assume the map germ $\pi\circ\widetilde{i}_{t_{0}}\colon(M,m_{0})\to(\Lambda_{2},\pi(z_{0}))$ is an embedding. Then the germ of its image surface possesses a canonical co-orientation in $\Lambda_{2}.$
The 3-manifold $\Lambda_{2}$ is non-orientable [Vassiliev1988], and ${\mathcal{Z}}$ is not oriented since $M$ is not. However, $M\times\{t_{0}\}$ is co-oriented in $M\times[0,1]$ by the positive $t$-direction, and, according to the lemma, the family of the maps $\pi\circ\widetilde{i}_{t}$ will allow us to compare the two co-orientations, and thus obtain the sign we are looking for.
Proof of the lemma.
The Lagrangian grassmanian $\Lambda_{2}$ under consideration is that of the Lagrangian planes in $\mathbb{R}^{4}_{v,w,V,W}$ with the symplectic form $d\alpha=dv{\wedge}dV+dw{\wedge}dW.$ The vertical plane is $v=w=0.$ Any Lagrangian plane transversal to the $vw$-coordinate plane is a graph of a linear map with a symmetric matrix
| $\left[\begin{array}[]{c}v\\ w\end{array}\right]=-\left[\begin{array}[]{c@{\quad}c}a\quad&b\\ b\quad&c\end{array}\right]\left[\begin{array}[]{c}V\\ W\end{array}\right].$ |
The entries $a,b,c$ are local coordinates on $\Lambda_{2}$ near the vertical point which is the origin in the chart. If $dV{\wedge}dW$ is an orientation of the vertical plane then we shall take $da{\wedge}db{\wedge}dc$ for a local orientation on $\Lambda_{2}.$ Swapping $V$ and $W$ swaps $a$ and $c$ to keep the matrix symmetric, and therefore reversal of the orientation of the vertical plane reverses the local orientation of $\Lambda_{2}.$ On the other hand, an orientation of the vertical plane induces, via the immersion map $i_{t_{0}}$, an orientation on $M$ near $m_{0}$. The latter induces in its own turn, via the embedding germ $\pi\circ\widetilde{i}_{t_{0}}$, an orientation on the surface $(\pi\circ\widetilde{i}_{t_{0}})(M)\subset\Lambda_{2}$ near the vertical point $(\pi\circ\widetilde{i}_{t_{0}})(m_{0})=\pi(z_{0}).$
We locally co-orient the image surface germ so that its co-orientation followed by its local orientation coming from $dV{\wedge}dW$ on the vertical plane gives the local orientation $da{\wedge}db{\wedge}dc$ on $\Lambda_{2}.$ $\square$
Example 4.2.
We rewrite the $D_{4}^{\pm}$ generating families of hypersurfaces given at the end of Sect. 3.2 as $u=F(x,y,\lambda)+vx+wy.$ In the local coordinates of Sect. 2.1, each of the two families of Legendrian maps is $(x,y,\lambda)\mapsto(u,v,w,V,W)\mapsto(u,v,w),$ where
| $v=-F_{x},\,\,w=-F_{y}\quad{\rm and}\quad V=(F+vx+wy)_{v}=x,\,\,W=(F+vx+wy)_{w}% =y.$ |
The Lagrangian planes tangent to the surfaces are
| $\left[\begin{array}[]{c}v\\ w\end{array}\right]=-\left[\begin{array}[]{c@{\quad}c}F_{xx}\quad&F_{xy}\\ F_{xy}\quad&F_{yy}\end{array}\right]\left[\begin{array}[]{c}V\\ W\end{array}\right]=-\left[\begin{array}[]{c@{\quad}c}\pm 2y\quad&\pm 2x\\ \pm 2x\quad&6y-2\lambda\end{array}\right]\left[\begin{array}[]{c}V\\ W\end{array}\right].$ |
According to Lemma 4.1, taking the orientation $dV{\wedge}dW$ on the vertical plane $v=w=0$ (that is, the local orientation $dx{\wedge}dy$ on $M$) we should take the orientation $da{\wedge}db{\wedge}dc\simeq dx{\wedge}dy{\wedge}d\lambda$ on $\Lambda_{2}.$ Thus, the co-orientation of $M\times\{0\}$ in $M\times\mathbb{R}_{\lambda}$ by the positive $\lambda$-direction is being sent to the canonical co-orientation of the germ $(\pi\circ\widetilde{i}_{0})(M)$ in $\Lambda_{2}.$ Therefore, in our $D_{4}^{\pm}$ bifurcations, the local intersection numbers $\langle{\mathcal{V}},{\mathcal{Z}}\rangle$ are 1.
The intersection number $\langle{\mathcal{V}},{\mathcal{Z}}\rangle,$ calculated as the sum of the individual $\pm 1$ contributions across all points of ${\mathcal{V}}\cap{\mathcal{Z}},$ is an invariant of oriented paths in the space ${\mathcal{L}}(M,N)$ connecting maps $\rho\circ i_{0}$ and $\rho\circ i_{1}.$ In Sect. 5.4 we will show that the sum $D_{4}^{+}+D_{4}^{-}$ is a cycle in ${\mathcal{L}}.$ Therefore, the number $\langle{\mathcal{V}},{\mathcal{Z}}\rangle$ is preserved in homotopies of paths with fixed ends. Existence of a well-defined local invariant $I_{\Sigma^{2}}$ on ${\mathcal{L}}$ for which
| $I_{\Sigma^{2}}(\rho\circ i_{1})=I_{\Sigma^{2}}(\rho\circ i_{0})+\langle{% \mathcal{V}},{\mathcal{Z}}\rangle,\quad{\rm with}\;I^{\prime}_{\Sigma^{2}}=D_{% 4}^{+}+D_{4}^{-},$ |
is equivalent to vanishing of $\langle{\mathcal{V}},{\mathcal{Z}}\rangle$ on all non-contractible loops in ${\mathcal{L}}.$
4.2 Opposite Self-Tangencies
We turn to the possible mod2 local invariant $I_{opp}$ of Sect. 2.4.
Opposite self-tangencies of fronts occur exactly at three types of the $TA_{1}^{2}$ strata: $TA_{1}^{e,0},$ $TA_{1}^{e,2}$ and $TA_{1}^{h,0}.$ In our proof of Theorem 2.6 in Sect. 5.4, we will show that the sum $TA_{1}^{2,opp}$ of these strata is a mod2 discriminantal cycle. We now give an example of a situation when this cycle is trivial.
Let $j$ be the involution of the space $ST^{*}\!N$ of framed contact elements of a manifold $N$ which changes the framing of each element to the opposite. An embedded Legendrian surface $i(M)\subset ST^{*}\!N$ has its antipodal surface $(j\circ i)(M).$
Assume $M$ is orientable and $N=\mathbb{R}^{3}.$ Take a direction in $\mathbb{R}^{3}$ and shift $(j\circ i)(M)$ along all vectors of this direction producing a semi-infinite cylinder ${\mathcal{C}}\subset ST^{*}\mathbb{R}^{3}$ parametrised by $M\times[0,\infty)$ and having the boundary $(j\circ i)(M).$ Choosing orientations on $ST^{*}\mathbb{R}^{3}$ and $M$, and taking the orientation on ${\mathcal{C}}$ so that $\partial C=-(j\circ i)(M),$ we obtain the integer intersection number $\ell_{opp}(i(M))=\langle{\mathcal{C}},(j\circ i)(M)\rangle.$ It does not depend on the choice of a direction in $\mathbb{R}^{3}$ and on orientation of $M.$
In generic homotopies of Legendrian surfaces, the value $\ell_{opp}(i(M))$ changes only at opposite self-tangencies of fronts—such moments correspond to intersections of a Legendrian surface with its antipodal surface. The increments are easily shown to be $\pm 2$ (see for example [Goryunov1997]). Therefore, the derivative of the mod2 local invariant $I_{opp}(\rho\circ i):=\ell_{opp}(i(M))/2$ is $TA_{1}^{2,opp}.$
5 Bifurcations in 2-Parameter Families of Fronts
Our proof of Theorems 2.3 and 2.6 is based on the study of bifurcations in generic 2-parameter families of fronts carried out in the first three subsections of this section. The bifurcation diagram of each family gives a linear equation on the increments of our local invarinats across the codimension 1 strata: the equation states that the total increment along a small generic loop in ${\mathcal{L}}$ around the codimension 2 stratum must vanish. The whole system of these equations guarantees that the corresponding linear combination of codimension 1 strata is a discriminantal cycle in ${\mathcal{L}}.$
We denote the increment across a particular stratum as the stratum itself, but in small characters. Increments which may be non-zero only in the mod2 case will be kept in square brackets, as well as non-co-orientable strata in formulas (but not in figures).
5.1 Gluing Codimension 1 Strata Together
We start with all 35 strata. Our initial goal is to reduce the number of unknown increments.
5.1.1 Extra $A_{1}$ Component
We begin with the easiest kind of codimension 2 bifurcations when an extra generic $A_{1}$ sheet of a front passes through a point of a codimension 1 bifurcation $S$. For cases 1–4 of the table below, the planar discriminants are of the shape shown in Fig. 5, left. Such a discriminant gives the equation $u=v$ for the increments. The discriminant for case 5 is in the same figure in its right.
The equations obtained at this stage allow us to consider in what follows sums of the strata differing only in some indices in their notation as introduced in Sect. 3.3. The notation of the increments across such bigger strata will follow the notational pattern for the elementary strata. If one of the summands in a big stratum is non-co-orientable, then the increment of any integer invariant across the big stratum is zero, and the big stratum is treated as non-co-orientable.
| $S$ | Equation | Big stratum | ||
|---|---|---|---|---|
| 1. | $TA_{1}^{3,r}$ | $r=2,3$ | $[a_{1}^{4,2}]=a_{1}^{4,3}=a_{1}^{4,4}$ | $\left[A_{1}^{4}\right]$ |
| 2. | $TA_{1}^{2,e,r}$ | $r=0,1,2$ | $ta_{1}^{3,r+1}=ta_{1}^{3,r}$ | $TA_{1}^{3}$ |
| 3. | $TA_{2}A_{1}^{e,\sigma}$ | $\sigma=\pm$ | $a_{2}a_{1}^{2,+,\sigma}=a_{2}a_{1}^{2,-\sigma,+}$ | |
| $a_{2}a_{1}^{2,-,\sigma}=a_{2}a_{1}^{2,-\sigma,-}$ | $A_{2}A_{1}^{2}$ | |||
| 4. | $A_{3}^{e,\sigma}$ | $\sigma=\pm$ | $a_{3}^{\sigma}a_{1}^{+}=a_{3}^{\sigma}a_{1}^{-}$ | |
| 5. | $A_{4}^{+}$ | $a_{3}^{+}a_{1}^{+}=a_{3}^{-}a_{1}^{-}$ | $A_{3}A_{1}$ | |
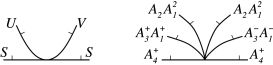
5.1.2 Cubic Bifurcations
According to Sect. 3.2, a generating family of hypersurfaces for the codimension one $A_{3}^{e,+}$ front singularity may be taken in the local normal form $u=x^{4}+v^{2}x^{2}+wx.$ Writing $v^{3}$ instead of $v^{2}$, we obtain a codimension 2 uni-germ singularity, with a versal deformation $u=x^{4}+(v^{3}+\lambda_{1}v+\lambda_{2})x^{2}+wx$ (see [Goryunov1983]; [Zakalyukin1983]). Its discriminant is a semi-cubical parabola $4\lambda_{1}^{3}+27\lambda_{2}^{2}=0$, and yields coincidence of the increments across its half-branches. Similarly replacing quadratic configurations by cubic in some other codimension 1 bifurcations $S$, we obtain a list like in the previous subsection:
| $S$ | Equations | Big stratum | |
|---|---|---|---|
| 6. | $A_{3}^{e,\sigma},\sigma=\pm$ | $a_{3}^{e,\sigma}=a_{3}^{h,\sigma}$ | $A_{3}^{e/h,\sigma}=A_{3}^{e,\sigma}+A_{3}^{h,\sigma}$ |
| 7. | $TA_{2}A_{1}^{e,\sigma},\sigma=\pm$ | $ta_{2}a_{1}^{e,\sigma}=ta_{2}a_{1}^{h,-\sigma}$ | |
| 8. | $TA_{1}^{2,e,r},TA_{1}^{2,h,r}$ | $ta_{1}^{2,e,2}=-ta_{1}^{2,e,0}=ta_{1}^{2,h,0}$ | $TA_{1}^{2,opp}=TA_{1}^{2,e,2}-TA_{1}^{2,e,0}+TA_{1}^{2,h,0}$ |
| $ta_{1}^{2,e,1}=[ta_{1}^{2,h,1}]$ | $[TA_{1}^{2,dir}]=TA_{1}^{2,e,1}+[TA_{1}^{2,h,1}]$ | ||
5.2 Particular Multi-Germ Families
So far we have reduced the number of unknown increments down to 17, out of which 2 may be non-trivial only mod2.
5.2.1 Non-Transversal Interaction with a Cuspidal Edge
We now consider cases of a cuspidal edge of a front ${\mathcal{F}}$ meeting other components of ${\mathcal{F}}$, but in a more degenerate manner at the meeting point than it happens in stable one-parameter bifurcations. Figure 6 illustrates three codimension 2 events of this kind when the plane tangent to ${\mathcal{F}}$ at its edge point is in a special position with another local component of ${\mathcal{F}}$:
-
•
the plane coincides with the plane tangent to a smooth $A_{1}$ sheet;
-
•
the plane contains the tangent direction of the line of intersection of two $A_{1}$ sheets;
-
•
the plane contains the tangent direction of another cuspidal edge.
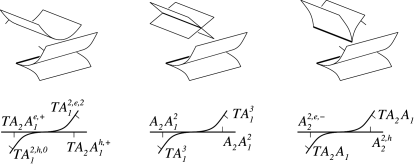
Respectively, we obtain new equations for the increments (the second equations in cases 9 and 11 arise when the framing of one of the components in Figure 6 is changed to the opposite):
| $\begin{array}[]{r@{\quad}cclcl}{\bf 9.}\quad&2ta_{1}^{2,opp}&=&ta_{2}a_{1}^{e,% +}-ta_{2}a_{1}^{h,+}\\ \quad&0&=&ta_{2}a_{1}^{e,-}-ta_{2}a_{1}^{h,-}\\ {\bf 10.}\quad&2ta_{1}^{3}&=&2a_{2}a_{1}^{2}\\ {\bf 11.}\quad&2ta_{2}a_{1}&=&a_{2}^{2,e,-}+a_{2}^{2,h}\\ \quad&&=&a_{2}^{2,e,+}-a_{2}^{2,h}\\ \quad\end{array}$ |
Equations 7 and 9(2) allow us to introduce a big stratum $TA_{2}A_{1}$ (which we are using already in 11). After this, 9(1) implies that the increment $ta_{1}^{2,opp}$ may be non-zero in the mod2 case only.
5.2.2 Interaction with a Swallowtail
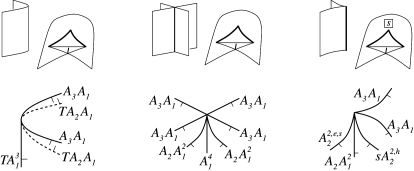
Figure 7 shows the events involving swallowtails. In the first case, at the most degenerate moment, the direction of the self-intersection curve at the swallowtail point is tangent to the incoming smooth sheet. The two other cases are clear.
The bifurcations provide the following equations:
| $\begin{array}[]{r@{\quad}cclcl}{\bf 12.}\quad&2a_{3}a_{1}&=&2ta_{2}a_{1}+ta_{1% }^{3}\\ {\bf 13.}\quad&[a_{1}^{4}]&=&0\\ {\bf 14.}\quad&2a_{3}a_{1}&=&a_{2}a_{1}^{2}+a_{2}^{2,e,+}-a_{2}^{2,h}\\ \quad&&=&a_{2}a_{1}^{2}+a_{2}^{2,e,-}+a_{2}^{2,h}\\ \quad\end{array}$ |
5.3 Uni-Germs of Codimension 2
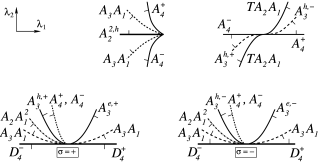
The three 2-parameter deformations represented in Fig. 8 are of generating families induced from versal deformations of $A_{5},A_{4}$ and $D_{5}$ function singularities: the top left, top right and two bottom bifurcation diagrams are respectively of the families
| $\begin{align}u &= &x^{6}+(\lambda_{1}\pm v+\gamma w)x^{4}+\lambda_{2}x^{3}+vx^{2}+wx,\quad% \gamma\in\mathbb{R};\\ u &= &x^{5}+vx^{3}+(\pm v^{2}+\lambda_{1}v+\lambda_{2})x^{2}+wx;\\ \sigma u &= &x^{2}y+y^{4}+\lambda_{1}y^{3}+\lambda_{2}y^{2}+vy+wx,\quad\sigma=\pm.\end{align}$ |
In the $A_{5}$ case, the generating family is the most generic. In the $A_{4}$ case, it has codimension 1 within all families induced from $A_{4}$. Similar $D_{4}$ degenerations do not occur since they would correspond to a change of topology of the source surface $M.$
The local normal form of the $A_{4}$ family was obtained in [Goryunov1983], and the one for the uni-modal $A_{5}$ family may be found by very similar methods.
In the bottom row of Figure 8, the $\lambda_{1}=\lambda_{2}=0$ fronts are front singularities of infinite codimension. Moreover, the 2-parameter deformations chosen are of infinite codimension in the space of all 2-parameter deformations of these fronts: indeed, the $A_{4}^{+}$ and $A_{4}^{-}$ strata of the bifurcation diagrams coincide. However, the only difference of our bifurcation diagrams with those of generic 2-parameter families of finite-codimensional singularities defined by generating families with the same principal parts is a split of the two $A_{4}$ strata.
Figure 8 gives the following equations:
| $\begin{array}[]{r@{\quad}rclclcl}{\bf 15.}\quad&a_{2}^{2,h}&=&a_{4}^{+}-a_{4}^% {-}\\ {\bf 16.}\quad&2ta_{2}a_{1}&=&a_{4}^{+}+a_{4}^{-}-a_{3}^{e/h,+}-a_{3}^{e/h,-}% \\ {\bf 17.}\quad&d_{4}^{+}-d_{4}^{-}&=&a_{4}^{+}+a_{4}^{-}-2a_{3}^{e/h,+}-2a_{3}% a_{1}+a_{2}a_{1}^{2}\\ \quad&d_{4}^{-}-d_{4}^{+}&=&a_{4}^{+}+a_{4}^{-}-2a_{3}^{e/h,-}-2a_{3}a_{1}+a_{% 2}a_{1}^{2}\\ \quad\end{array}$ |
5.4 Proofs of Theorems 2.3 and 2.6
One can show that any further generic 2-parameter families of fronts (for example, passage of an extra $A_{1}$ sheet through a quadruple or $D_{4}$ bifurcation, or taking other framing options in Fig. 6, left) are not able to provide us with any equations on the increments independent from those we have already obtained.
We collect Eqs. 9–17 into two tables below, for arbitrary and mod2 coefficients. Each of the two tables consists of two parts. The left one contains the equations themselves in the order they have appeared (we are using dots for zero coefficients), while the right lists the discriminantal cycles as linear combinations of the strata (with the notations as in Lemma 3.1). The bars in the first table indicate the equations which are integer linear combinations of the others.
| 9 | $\overline{10}$ | 11 | 11 | 12 | 13 | 14 | $\overline{14}$ | 15 | 16 | 17 | $\overline{17}$ | $I^{\prime}_{s}/2$ | $\widehat{I^{\prime}}_{s}/2$ | $I^{\prime}_{c}/2$ | $\widehat{I}^{\prime}_{c}/2$ | $I^{\prime}_{t}$ | $I^{\prime}_{\Sigma^{2}}$ | $I^{\prime}_{0}$ | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| $[A_{1}^{4}]$ | . | . | . | . | . | 1 | . | . | . | . | . | . | . | . | . | . | . | . | . | |
| $TA_{1}^{2,opp}$ | 2 | . | . | . | . | . | . | . | . | . | . | . | . | . | . | . | . | . | . | |
| $[TA_{1}^{2,dir}]$ | . | . | . | . | . | . | . | . | . | . | . | . | . | . | . | . | . | . | . | |
| $TA_{1}^{3}$ | . | 2 | . | . | 1 | . | . | . | . | . | . | . | . | . | . | . | 2 | . | . | |
| $A_{2}A_{1}^{2}$ | . | $-$2 | . | . | . | . | 1 | 1 | . | . | 1 | 1 | . | . | . | . | 2 | . | . | |
| $A_{2}^{2,e,+}$ | . | . | . | 1 | . | . | 1 | . | . | . | . | . | . | . | 2 | 2 | . | . | 1 | |
| $A_{2}^{2,e,-}$ | . | . | 1 | . | . | . | . | 1 | . | . | . | . | . | . | 2 | $-2$ | . | . | $-1$ | |
| $A_{2}^{2,h}$ | . | . | 1 | $-$1 | . | . | $-$1 | 1 | $-$1 | . | . | . | . | . | . | 2 | . | . | 1 | |
| $A_{3}A_{1}$ | . | . | . | . | $-$2 | . | $-$2 | $-$2 | . | . | $-2$ | $-2$ | . | . | 1 | . | 1 | . | . | |
| $TA_{2}A_{1}$ | . | . | $-$2 | $-$2 | 2 | . | . | . | . | $-$2 | . | . | . | . | 1 | . | . | . | . | |
| $A_{3}^{e/h,+}$ | . | . | . | . | . | . | . | . | . | $-$1 | $-2$ | . | 1 | 1 | . | . | . | . | 1 | |
| $A_{3}^{e/h,-}$ | . | . | . | . | . | . | . | . | . | $-$1 | . | $-2$ | 1 | $-1$ | . | . | . | . | . | |
| $A_{4}^{+}$ | . | . | . | . | . | . | . | . | 1 | 1 | 1 | 1 | 1 | . | 1 | 1 | . | . | 1 | |
| $A_{4}^{-}$ | . | . | . | . | . | . | . | . | $-$1 | 1 | 1 | 1 | 1 | . | 1 | $-1$ | . | . | . | |
| $D_{4}^{+}$ | . | . | . | . | . | . | . | . | . | . | $-1$ | 1 | . | 1 | . | . | . | 1 | $-1$ | |
| $D_{4}^{-}$ | . | . | . | . | . | . | . | . | . | . | 1 | $-1$ | . | 3 | . | . | . | 1 | . | |
Here $I^{\prime}_{0}=\left(I^{\prime}_{s}/2+\widehat{I}^{\prime}_{s}/2+\widehat{I}^{% \prime}_{c}/2-3I^{\prime}_{\Sigma^{2}}\right)/2=\left(2I^{\prime}_{s_{+}}+I^{% \prime}_{c_{+}}-I^{\prime}_{c_{-}}-6I^{\prime}_{\Sigma^{2}}\right)/4.$
The rank count in the left part of the table shows that the space of integer discriminantal cycles is six-dimensional, and the right part of the table tells us that a basis of this integer space is formed by the six cycles excluding $\widehat{I}^{\prime}_{c}/2.$ This proves Theorem 2.3.
Adjusting the above table to the $\mathbb{Z}_{2}$ case, we use Eq. 11 to make a big stratum $A_{2}^{2.e/h}$, then exclude the $\left[A_{1}^{4}\right],$ $TA_{1}^{3}$ and $A_{2}A_{1}^{2}$ strata (along with the Eqs. 13, 12 and 14 in which exactly one of them appears now on its own), and drop the duplicate and $0=0$ equations. This leaves us with 3 independent equations in 11 unknowns:
| $\mathbb{Z}_{2}$ | 15 | 16 | 17 | $I^{\prime}_{s}/2$ | $\widehat{I}^{\prime}_{s}/2$ | $I^{\prime}_{c}/2$ | $\widehat{I}^{\prime}_{c}/2$ | $I^{\prime}_{t}$ | $I^{\prime}_{\Sigma^{2}}$ | $I^{\prime}_{opp}$ | $I^{\prime}_{dir}$ | $I^{\prime}_{0}$ |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| $TA_{1}^{2,opp}$ | . | . | . | . | . | . | . | . | . | 1 | . | . |
| $[TA_{1}^{2,dir}]$ | . | . | . | . | . | . | . | . | . | . | 1 | . |
| $A_{2}^{2,e/h}$ | 1 | . | . | . | . | . | . | . | . | . | . | 1 |
| $A_{3}A_{1}$ | . | . | . | . | . | 1 | . | 1 | . | . | . | . |
| $TA_{2}A_{1}$ | . | . | . | . | . | 1 | . | . | . | . | . | . |
| $A_{3}^{e/h,+}$ | . | 1 | . | 1 | 1 | . | . | . | . | . | . | 1 |
| $A_{3}^{e/h,-}$ | . | 1 | . | 1 | 1 | . | . | . | . | . | . | . |
| $A_{4}^{+}$ | 1 | 1 | 1 | 1 | . | 1 | 1 | . | . | . | . | 1 |
| $A_{4}^{-}$ | 1 | 1 | 1 | 1 | . | 1 | 1 | . | . | . | . | . |
| $D_{4}^{+}$ | . | . | 1 | . | 1 | . | . | . | 1 | . | . | 1 |
| $D_{4}^{-}$ | . | . | 1 | . | 1 | . | . | . | 1 | . | . | . |
The space of mod2 invariants has rank 8. There is one relation on the 9 discriminantal cycles listed in the table: $I^{\prime}_{s}/2+\widehat{I}^{\prime}_{s}/2+\widehat{I}^{\prime}_{c}/2+I^{% \prime}_{\Sigma^{2}}\equiv 0.$ Our proof of Theorem 2.6 is finished.
6 Non-Framed Fronts in Oriented 3-Manifolds
We now consider a natural simplification of our main problem: the fronts will not be framed. This corresponds to Legendrian maps $M\looparrowright PT^{*}\!N\to N,$ of immersed Legendrian submanifolds in the space of non-framed contact elements of $N.$ We denote the set of all such maps by ${\mathcal{L}}_{nf}={\mathcal{L}}_{nf}(M,N).$
As a result of the framing omission, the $A_{2}A_{1}$ and $A_{3}$ points of generic fronts lose their signs. Moreover, for our work with the space ${\mathcal{L}}_{nf}$ we get additional unions into big strata, and certain strata become non-co-orientable in ${\mathcal{L}}_{nf}$:
| $\displaystyle A_{2}^{2,e}=A_{2}^{2,e,+}+A_{2}^{2,e,-},\qquad A_{3}^{e/h}=A_{3}% ^{e/h,+}+A_{3}^{e/h,-},$ | |||
| $\displaystyle[TA_{1}^{2}]=[TA_{1}^{2,opp}]+[TA_{1}^{2,dir}],\qquad[D_{4}^{+}],% \qquad[D_{4}^{-}].$ |
The first table from Sect. 5.4 becomes the one below. Its right part gives a natural basis of the space of integer discriminantal cycles.
| $\overline{9}$ | $\overline{10}$ | 11 | 11 | 12 | 13 | 14 | $\overline{14}$ | 15 | 16 | $\overline{17}$ | $\overline{17}$ | $I^{\prime}_{s}/2$ | $I^{\prime}_{c}/2$ | $I^{\prime}_{t}$ | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| $[A_{1}^{4}]$ | . | . | . | . | . | 1 | . | . | . | . | . | . | . | . | . |
| $[TA_{1}^{2}]$ | . | . | . | . | . | . | . | . | . | . | . | . | . | . | . |
| $TA_{1}^{3}$ | . | 2 | . | . | 1 | . | . | . | . | . | . | . | . | . | 2 |
| $A_{2}A_{1}^{2}$ | . | $-$2 | . | . | . | . | 1 | 1 | . | . | 1 | 1 | . | . | 2 |
| $A_{2}^{2,e}$ | . | . | 1 | 1 | . | . | 1 | 1 | . | . | . | . | . | 2 | . |
| $A_{2}^{2,h}$ | . | . | 1 | $-$1 | . | . | $-$1 | 1 | $-$1 | . | . | . | . | . | . |
| $A_{3}A_{1}$ | . | . | . | . | $-$2 | . | $-$2 | $-$2 | . | . | $-$2 | $-$2 | . | 1 | 1 |
| $TA_{2}A_{1}$ | . | . | $-$2 | $-$2 | 2 | . | . | . | . | $-$2 | . | . | . | 1 | . |
| $A_{3}^{e/h}$ | . | . | . | . | . | . | . | . | . | $-$2 | $-$2 | $-$2 | 1 | . | . |
| $A_{4}^{+}$ | . | . | . | . | . | . | . | . | 1 | 1 | 1 | 1 | 1 | 1 | . |
| $A_{4}^{-}$ | . | . | . | . | . | . | . | . | $-$1 | 1 | 1 | 1 | 1 | 1 | . |
| $[D_{4}^{+}]$ | . | . | . | . | . | . | . | . | . | . | 1 | 1 | . | . | . |
| $[D_{4}^{-}]$ | . | . | . | . | . | . | . | . | . | . | 1 | 1 | . | . | . |
Thus we have
Proposition 6.1.
For any surface $M$ and oriented 3-manifold $N$, the space of integer local invariants of non-framed fronts in $N$ parametrised by $M$ has rank 3. Its basis is formed by half the numbers of swallowtail and $A_{2}A_{1}$ points, and the number of triple points of a front.
The $\mathbb{Z}_{2}$ version of the last table is on the next page, with the duplicate and $0=0$ equations omitted, and a solution basis on the right. We are using $I^{\prime}_{\ell}$ there since for $N=\mathbb{R}^{3}$ this discriminantal cycle integrates to the edge self-linking number $I_{\ell}$ of Sect. 2.3 reduced mod2. The meaning of $I_{\Sigma^{2}}$ for paths in ${\mathcal{L}}_{nf}$ here is similar to that for the integer framed case given in Sect. 4.1, with the projectivisation of $T^{*}\!N$ used now instead of its spherisation. The cycle $I^{\prime}_{tan}$ counts the parity of the number of self-tangencies of fronts along generic paths in ${\mathcal{L}}_{nf}.$
Hence we have obtained
Proposition 6.2.
For any surface $M$ and oriented 3-manifold $N$, the space of mod2 discriminantal cycles in ${\mathcal{L}}_{nf}(M,N)$ has rank 6, with a basis given in the above table. The space of $\mathbb{Z}_{2}$-valued local invariants in this case has at least rank 3 and at most 6.
| $\mathbb{Z}_{2}$ | 11 | 12 | 13 | 14 | 15 | 16 | 17 | $I^{\prime}_{s}/2$ | $I^{\prime}_{c}/2$ | $I^{\prime}_{\ell}$ | $I^{\prime}_{t}$ | $I^{\prime}_{\Sigma^{2}}$ | $I^{\prime}_{tan}$ |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| $[A_{1}^{4}]$ | . | . | 1 | . | . | . | . | . | . | . | . | . | . |
| $[TA_{1}^{2}]$ | . | . | . | . | . | . | . | . | . | . | . | . | 1 |
| $TA_{1}^{3}$ | . | 1 | . | . | . | . | . | . | . | . | . | . | . |
| $A_{2}A_{1}^{2}$ | . | . | . | 1 | . | . | 1 | . | . | . | . | . | . |
| $A_{2}^{2,e}$ | 1 | . | . | 1 | . | . | . | . | . | . | . | . | . |
| $A_{2}^{2,h}$ | 1 | . | . | 1 | 1 | . | . | . | . | . | . | . | . |
| $A_{3}A_{1}$ | . | . | . | . | . | . | . | . | 1 | . | 1 | . | . |
| $TA_{2}A_{1}$ | . | . | . | . | . | . | . | . | 1 | . | . | . | . |
| $A_{3}^{e/h}$ | . | . | . | . | . | . | . | 1 | . | . | . | . | . |
| $A_{4}^{+}$ | . | . | . | . | 1 | 1 | 1 | 1 | 1 | 1 | . | . | . |
| $A_{4}^{-}$ | . | . | . | . | 1 | 1 | 1 | 1 | 1 | 1 | . | . | . |
| $[D_{4}^{+}]$ | . | . | . | . | . | . | 1 | . | . | . | . | 1 | . |
| $[D_{4}^{-}]$ | . | . | . | . | . | . | 1 | . | . | . | . | 1 | . |
One can easily see that both propositions stay valid even if the 3-manifold is not oriented.
Remark 6.3.
Yet another option to consider is framed fronts in a non-oriented 3-manifold. Comparing with the tables of Sect. 5.4, this leads to the bigger strata $A_{2}^{2,e}$ and $A_{4}$, and to the $A_{2}^{2,h}$ stratum losing its co-orientation. As a result the rank of the space of integer discriminantal cycles reduces to 5 and of the mod2 space reduces to 7. This is due to the disappearance of the integer cycle $\widehat{I}^{\prime}_{c}/2$ and hence of the cycle $I^{\prime}_{0}$ for both coefficient choices.
References
- [Aicardi1997] Aicardi, F.: Discriminants and local invariants of planar fronts. In: Arnold, V.I., Gelfand, I.M., Retakh, V.S., Smirnov, M. (eds.) The Arnold–Gelfand Mathematical Seminars. Geometry and Singularity Theory, pp. 1–76. Birkhäuser, Boston (1997)
- [Arnold1976] Arnold, V.I.: Wave front evolution and equivariant Morse lemma. Commun. Pure Appl. Math. 29(6), 557–582 (1976)
- [Arnold et al.1985] Arnold, V.I., Gusein-Zade, S.M., Varchenko, A.N.: Singularities of differentiable maps. Vol. I. The classification of critical points, caustics and wave fronts. Monographs in Mathematics, vol. 82, pp. xi+382. Birkhäuser, Boston (1985)
- [Arnold1990] Arnold, V.I.: Singularities of caustics and wave fronts. Mathematics and its Applications (Soviet Series), vol. 62, pp. xiv+259. Kluwer, Dordrecht (1990)
- [Arnold1995] Arnold, V.I.: Invarianty i perestroiki ploskih frontov. In: Osobennosti gladkikh otobrazheniy s dopolnitel’nymi strukturami. Trudy Mat. Inst. Steklov. 209, 14–64 (1995). [English translation: Invariants and perestroikas of wave fronts on the plane. In: Singularities of smooth mappings with additional structures. Proc. Steklov Inst. Math. 209, 11–56 (1995)]
- [Goryunov1997] Goryunov, V.: Local invariants of mappings of surfaces into three-space. In: Arnold, V.I., Gelfand, I.M., Retakh, V.S., Smirnov, M. (eds.) The Arnold–Gelfand Mathematical Seminars. Geometry and Singularity Theory, pp. 223–255. Birkhäuser, Boston (1997)
- [Goryunov2013] Goryunov, V.: Local invariants of maps between 3-manifolds. J. Topol. 6, 757–778 (2013)
- [Goryunov1983] Goryunov, V.: Singularities of projections of complete intersections. Curr. Probl. Math. 22, 167–206 (1983). Itogi Nauki i Tekhniki, Akad. Nauk SSSR, VINITI, Moscow (Russian). [English translation: J. Sov. Math. 27(3), 2785–2811 (1984)]
- [Tchernov2002] Tchernov, V.: Arnold-type invariants of wave fronts on surfaces. Topology 41(1), 1–45 (2002)
- [Vassiliev1994] Vassiliev, V.A.: Cohomology of knot spaces. Theory of singularities and its applications. In: Advances in Soviet Mathematics, vol. 1, pp. 225–262. American Mathematical Society, Providence (1994)
- [Vassiliev1988] Vassiliev, V.A.: Lagrange and Legendre characteristic classes. In: Advanced Studies in Contemporary Mathematics, vol. 3, pp. x+268. Gordon and Breach Science Publishers, New York (1988)
- [Zakalyukin1983] Zakalyukin, V.M.: Reconstructions of fronts and caustics depending on a parameter, and versality of mappings. Curr. Probl. Math. 22, 56–93 (1983). Itogi Nauki i Tekhniki, Akad. Nauk SSSR, VINITI, Moscow (Russian). [English translation: J. Sov. Math. 27(3), 2713–2735 (1984)]